Understanding the language of mathematics plays a large role in students becoming numerate. Developing ways to use mathematical terminology, symbols and numerals is new for all of us at first, and may feel like learning a second (or third or fourth) language. The working mathematically processes of communicating and reasoning are at the heart of our NSW mathematics syllabus. These processes emphasise the need for students to be able to not only know the content, but to be able to share and explain how they understand the content. This requires students to be able to read and interpret mathematics when written in words, and to be able to make sense of mathematics through connecting language, pictures, symbols and in concrete experiences (Haylock and Cockburn, 1989). Student’s literacy skills therefore have the potential to impact their success in numeracy.
“… a large percentage of children fail mathematics because they can’t read nor understand the wording of the tasks they are given to solve by mathematics teachers.”
Excerpt from Biography of Anne Newman as a teacher
To support students for whom English is an Additional Language or Dialect (EAL/D), ACARA’s EAL/D Learning Progression annotations for mathematics is a helpful document that provides language considerations and teaching strategies for classroom teachers.
In mathematics, we often ‘borrow’ teaching strategies from literacy to implement in the mathematics classroom. This can be very effective. I have used strategies such as asking Here, Hidden, Head questions when interpreting and analysing graphs in data. The use of word maps (page 124, the good ol’ Programming and Strategies Handbook circa late 90s early 2000s) is useful in mathematics to develop student-made posters to explain concepts in the classroom. Also, the Frayer Model is easily adaptable for mathematics and is a visual representation I often use, it has particular benefits as it requires students to think deeply about the concept when identifying examples and non-examples.
There is one example though where taking a literacy strategy and applying it in mathematics context can be more problematic that helpful, that is, a key-word approach to word problems.
When we use a key-word approach in English, students generally learn to look out for the who, what, when, where or how. For example, it doesn’t matter whether I say “I went to the shops with my mum on Saturday” or “On Saturday mum and I went to the shops” you would still be able to understand the intent of the statement and answer questions about who went to the shops and when did they go and get the same answer.
This is a very different story in mathematics. In mathematics the order the words appear in a sentence matters and it’s actually the smaller words- prepositions and connectives, that matter most. For example, if I said, “Take five from seven” or “Take seven from five”, the order of the words matters, producing two different solutions. Or if I said, “Altogether Blake and Zoe had 29 blue marbles, how many marbles could they have each?” The important words are ‘and’, ‘altogether’, ‘could’ and ‘each’. If a student applies a key-word strategy they may recall from English, they would most likely highlight or circle Blake, Zoe and marbles. These words provide the context for the problem but do not give any useful information to assist the student in solving the problem. It’s those smaller words that matter.
In mathematics it is therefore better to leave the whole problem as-is and ask students to write what the problem is asking them to do or find out? Students may need to read and re-read the question a number of times to make sure they understand the problem, even our most capable students make errors when reading mathematics problems and sometimes this may be due to the nature of how we read for meaning, by skimming and scanning.
Anne Newman’s research with word-based problems in mathematics led her to develop the Newman’s Error Analysis. This is a technique that is now widely used in many schools in assisting students to solve mathematical word problems and to assist teachers in identifying where students may be having difficulty or struggling. Anne talked about that sometimes when students get answers to say division questions incorrect, the teacher would give the student more division questions. When in fact, the student may have been struggling with the written aspect of the problem, say the reading or comprehension of the problem. Therefore a focus on language was needed (see below for links to resources for Newman’s Error Analysis).
In my blog Let’s get into shape! I discussed a number of language issues within the area of Two- and Three- Dimensional Space. There are a few more language complexities that often come up in discussions I’ve had with teachers around mathematics. As teachers, it is impossible to always get the language correct, I still catch myself sometimes using the word ‘times’, as in, six times four when I should say six multiplied by four or six fours. I don’t want to become the semantics police but there are a number of instances when the ‘correct’ terms are essential as they express meaning and some misunderstandings students develop are based on language.
Some common points regarding language in mathematics:
- 2D is shape and 3D is object
In Australia we use the word shape when talking about Two-Dimensional Space and object when talking about Three-Dimensional Space. When you just use the word shape for both, students may not develop the understanding that they are different. It is also helpful to use the word object as you can pick up Three-Dimensional objects. It is also good to note that in Stage 4 in NSW students are introduced to the word solid to represent objects.
- Does a cone have an apex or vertex?
Good question! Isn’t it nice when the problematic nature of definitions in mathematics creates a deep debate or conversation! This is a discussion that could go around in circles and I guess that’s why I mention it here. There are so many different definitions and they are still evolving and in some cases the definitions first written were quite simple and have become differentiated over time. An apex is the name for a specific vertex on an object (apex as the highest ‘point’ opposite the base). So technically it could be either an apex or a vertex, our syllabus states it as a vertex, so I’d go with that.
- They are sector graphs not pie charts
In other countries they may be referred to as pie charts but in NSW we use the term sector graph. I think it is more appropriate as one; when representing data in a circle, the fractional parts are called sectors therefore it matches the part of the circle you are referring to. Two; for any students for whom English is an additional language or dialect, pie chart may not make sense due to the everyday use of the word pie. If you are teaching in NSW, we specifically mention sector graphs in Stage 4 of the syllabus. In our previous syllabus (2002 edition) sector graphs were introduced in Stage 3. This means that in mathematics, graphs using percentages (divided bar graphs and sector graphs) are not introduced until Stage 4. You may still like to show sector graphs as examples of data for discussions or for explaining uses of percentages in Stage 3 but there is no outcome that states students need to be able to read, interpret or create them. There is information on the numeracy skills framework website to assist with graphs.
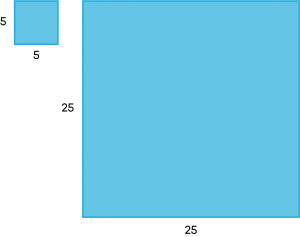
- It’s square metres not metres squared
This is probably one of the more important ones to get right as these two terms have very different meanings. With any unit of measure for area (cm, m, km etc) we need to say square metres. So, if it’s 25 m2 you read this as “25 square metres.” This then tells the audience that the shape is made up of 25 ‘squares’ for example, it could have the dimensions 5 m x 5 m or 2 m x 12.5 m. If I read it as “25 metres squared” this squares the 25 metres, so the shape would be 25 m x 25 m which would have a different total area.
- It’s one-quarter not one over four
Maybe I should have placed this at number one! When referring to fractions it is important to not use whole number language. It’s one-quarter or two-fifths or seven-eighths. We want students to understand that fractions are numbers, not two separate numbers. It is also important to focus on the written words before introducing students to the symbolic forms. So spend plenty of time in Kindergarten, Year 1 and Year 2 writing the fractions in words (both the teacher and student) when recording so students develop the language associated with fractions that they can then attached the symbols to.
- It’s mass not weight
Colloquially, your mass is often referred to as your weight. This is an everyday use of the word and can be discussed in class. Mathematically though, you are measuring mass (in grams or kilograms), as weight is related to the amount of force acting upon an object and is generally due to gravity and is measured in newtons. Class discussions on mass and weight can be explored with Stage 2 or 3 students if/ when you are talking about space (e.g. moon’s gravity).
- It’s “five point seven five” not “five point seventy-five”
Similarly to the comments on fractions, when talking about decimals avoid referring to the digits after the decimal as whole numbers. This may lead to students having the misunderstanding that there are whole numbers on both sides of the decimal. For 5.75 say “five point seven five” or “five and seventy-five hundredths.”
For further advice on when to introduce students to specific mathematical language refer to the Language section on each content page of the mathematics K-10 syllabus. Any new words that are introduced will be in bold in the language section.
Resources
Language in the Mathematics Classroom The Digest, NSW Institute of Teachers, ACER
Frayer model in mathematics Extending Vocabulary- The Frayer Model Ontario Association for Mathematics Education, Canada.
Example videos for Newman’s Error Analysis from The Numeracy Skills Framework
A Revaluation of Newman’s Error Analysis by Allan White, UWS
Active mathematics in classrooms: finding out why children make mistakes – and then doing something to help them Allan L. White, UWS
The Influences of Different Number Languages on Numeracy Learning Canadian Language and Literacy Research Network
The role of language in mathematics National Association for Language Development in the Curriculum , UK.
Mind your language: Speaking in and about the mathematics classroom David Clarke , University of Melbourne
Literacy, Language and Mathematics Learning canberramaths.org.au
References
Haylock, D., & Cockburn, A. (1989). Understanding early years mathematics. London: Paul Chapman.
Newman, M. A. (1977). An analysis of sixth-grade pupils’ errors on written mathematical tasks. Victorian Institute for Educational Research Bulletin, 39, 31-43.
NSW Education Standards Authority (NESA). (2012). Mathematics K-6 syllabus. Sydney, NSW.: Author.