Noticing the mathematics: Making the connections Part 2

Making learning more robust

Peter Sullivan delivered the opening keynote on Saturday morning. Peter talked about the importance of connecting student learning with curriculum goals and subsequent learning experiences to make learning more robust and transferable. Students’ learning needs to be activated – but then what? Peter posed the question what does consolidating look like? Students should think for themselves and engage in actively creating their own knowledge. For this to occur, tasks need to be structured to maximise engagement and then these tasks need to be sequenced so the lessons build on each other. This is what effective curriculum planning looks like.

Effective programming involves teachers being active prior, during and after the lesson in planning (and being ready for) students’ responses. Peter referred to Smith and Stein’s work on the five practices for orchestrating productive mathematics discussions (David Butler @DavidKButlerUoA wrote a great review blog you can read here).

Smith and Stein’s work focuses on the planning that happens prior to the lesson, the monitoring and facilitating that occurs during the lesson and the role of the teacher in drawing connections between students’ responses as well as connecting their responses to the mathematical concepts. Peter added two more practices – sharing students’ responses and encouraging students to learn by listening to others (a nice link to talk moves).

Peter went on to discuss the role students play in their own learning. They need to study exemplars and models to prompt further thinking and self-improvement. Students can then take ideas and adapt them, extending their thinking. Peter said too many students’ thinking seems to become more routinised rather than flexible. While teachers help students make sense of the mathematics, the hard work needs to be done by the students. Peter said that tasks should ideally:

Students should have opportunities to explore tasks without always having explicit instructions from the teacher up front. if the teacher says ‘this is how you do it’, then whatever the student does is just a reproduction of what the teacher knows – where is the learning? where is the new knowledge? Peter supported the idea of using a launch, explore, summarise teaching model (one I have blogged about in the past). He encouraged teachers to try it out in the classroom and learn from the students, from their responses – plan to notice. Peter then shared a number of tasks that provide challenge for all students and demonstrated some good questioning techniques to use during the lesson. Allowing students to explore a task doesn’t negate explicit teaching, it just moves it to a more appropriate place in the lesson – which is often after students have attempted and struggled (productively) with the task first.

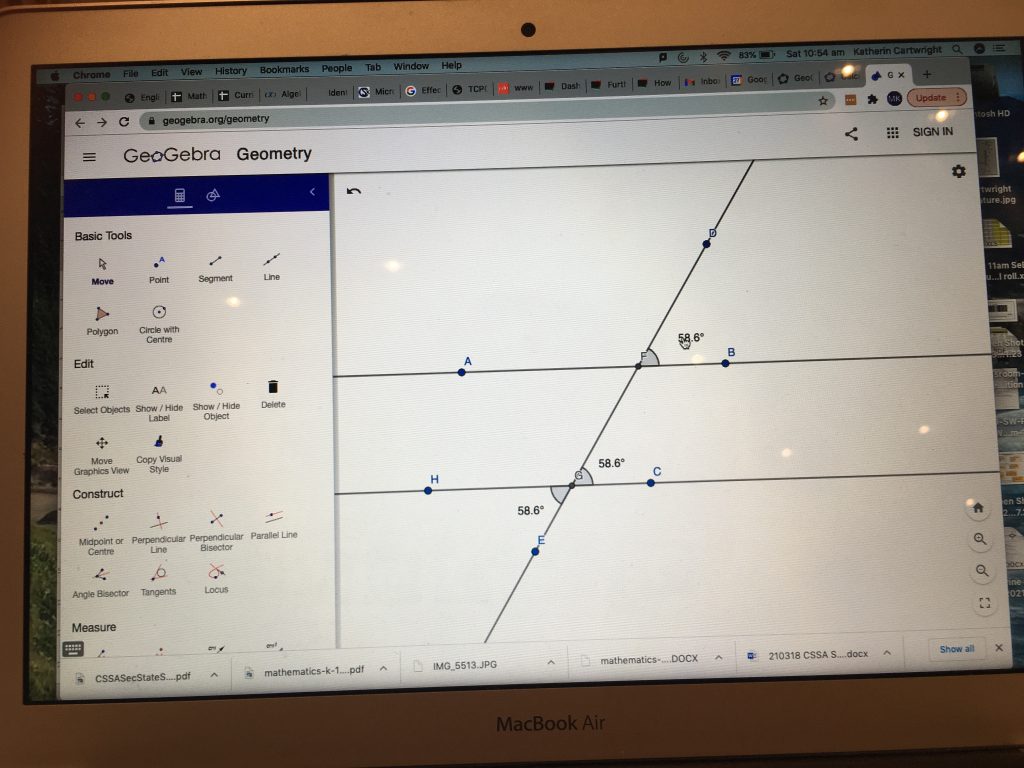
GeoGebra – Learning through discovery

Motion mathematics

I also attended Associate Professor Jenni Way’s (@Way8Jennifer) workshop session on young students’ representations – specifically the use of gesture. Jenni talked about how to use body movement to help students notice what we want them to notice (mathematically). She talked about the power of learning by doing and how mathematics could gain some ideas from other areas such as sport – tasks that require physicality. Improving at sport often focuses on high expectations, patience, and practicing parts of the whole.

Jenni had us creating shapes with string and masking tape and had us working in groups – during the group work all participants used gesture to collectively work out how to solve the task. Jenni shared that students, just like we did, often spontaneously use gesture when talking about shapes. She went on to mention that when students have less or limited vocabulary, more gesture can be noticed. This was something to think about further when working with students who’s mathematical vocabulary is still developing or is limited. Gesture is one way students may be able to communicate understanding and knowledge that hasn’t been noticed before. Recent research by Jenni and her colleague Christine Preston also revealed that students’ gesture showed a greater level of conceptual understanding than through their verbal responses or drawings. Jenni challenged us to consider – what do you already use gesture for? and what new ideas do you have?

There were plenty more workshops that I wasn’t able to attend (some pictured below), i love that each year the calibre of workshops continue to grow and there is never enough time to see them all! As I mentioned in my previous blog, you can also check out the hashtag #noticingthemaths to see more of what went on at the conference. I’m already looking forward to 2022! Thanks MANSW and the PAM committee team.