As students start to return to school one day a week and then full-time, teachers will be spending much of this time re-assessing what students know and planning future teaching based on where to next. When it comes to mathematics, identifying students’ prior knowledge is essential as mathematics concepts build upon each other. Identifying key concepts, or big ideas is a way to try and avoid ‘gaps’ in students’ learning and development.
Instead of focusing on smaller concepts, it can be beneficial to broaden the scope of teaching and learning in mathematics to focus on big ideas. When we do this, it allows time for students to ‘play’ and ‘explore’ the mathematics and also to see the connections between mathematical concepts. One way of doing this is to cluster groups of content from the curriculum together under big ideas. Grouping concepts into clusters also allows for differentiation:
“Overall curriculum expectations are likely to provide a starting point for what the key concepts are, but probably do not help a teacher easily plan to differentiate instruction. One approach is to cluster specific curriculum expectations and use them as learning goals over a series of lessons. It is by clustering specific expectations, in conjunction with looking at the curriculum for other grade levels, that the key concepts become evident.”
In NSW, the NSW Education Standards Authority (NESA) made an announcement in April about changes to the K-10 curriculum requirements for 2020 due to coronavirus:
The flexibility that this decision allows teachers is welcomed and provides time for teachers to focus on the essential content for students. Although concepts may differ for individual students, I thought it would be helpful to suggest a few ‘big ideas’ that might be a starting point for the rest of the year. I had previously created stage-based content clusters to assist teachers in planning using big ideas, and these might be useful in this process. They were created based on NSW syllabus outcomes and key ideas (as this is my teaching background). However, the clustering and the concept statements I have developed for each cluster, would also be useful ways to group Australian Curriculum descriptors, or curriculum content from other countries.
Within this blog I have selected two content clusters that I believe would be good to focus on in particular (or to start with). Each of these clusters have links to curriculum across all year grades of primary schooling so whether you are teaching a straight year class, a composite, or multi-stage class, the concept is still the same – it just develops in sophistication. As much of the rich network of connections in mathematics are founded in number concepts, I have selected a cluster related to partitioning and part-whole number knowledge, and a cluster related to relationships, specifically fractions. This is not to say you couldn’t/ shouldn’t focus on other outcomes and content outside these clusters. They are just ones that other concepts often build and develop from so it might be a good place to start.
Partitioning
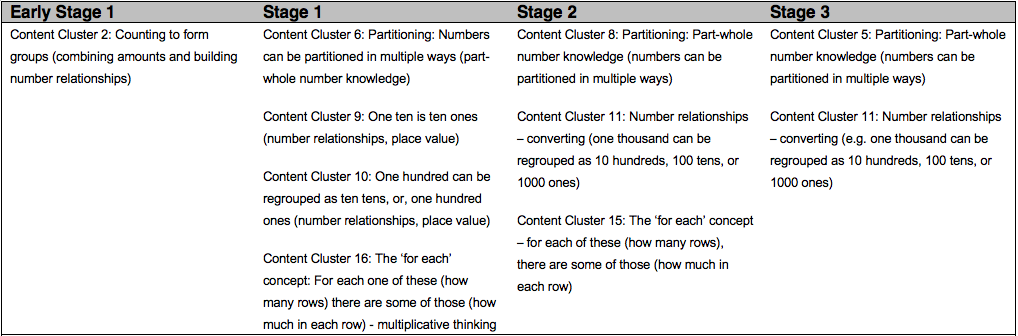
The first big idea is partitioning. Students in all grades develop their understanding of partitioning as they break numbers into smaller parts, as they compare numbers, as they learn how the parts relate to the whole, and as they see how partitioning links to patterns and the structure of number. If students have gaps in understanding how to partition, this impacts on their understanding of place value, fractions, the four operations and their related strategies, and applying partitioning to understand units of measurement. I have developed clusters for each stage of learning that deal with partitioning, this is not an exhaustive list! The following image shows clusters from each stage that are all related to a similar aspect of partitioning.
For the clusters I have developed in this group there are links to the following substrands:
- Early Stage 1 (Kindergarten): addition and subtraction, multiplication and division, patterns and algebra
- Stage 1 (Years 1 and 2): whole numbers, addition and subtraction, multiplication and division, fractions and decimals, patterns and algebra, length
- Stage 2 (Years 3 and 4): whole numbers, multiplication and division, fractions and decimals, length, area, volume, mass
- Stage 3 (Year 5 and 6): whole numbers, multiplication and division, fractions and decimals, length, volume and capacity, mass
In my planning, I would select a cluster, if I was teaching Year 1, I might choose Cluster 6: Partitioning: Numbers can be partitioned in multiple ways (part-whole number knowledge). The cluster (image below) deals with a number of outcomes and key ideas. There is not set time for how long to spend on teaching the concept in the cluster. It might take me three weeks, it might be only two. These are decisions that need to be made by the teacher based on students’ needs, abilities and interests. You could focus on each outcome (e.g. multiplication and division) for three lessons, or your lessons might actually blend across the outcomes. However you choose to teach the smaller key ideas within this cluster, the focus will always come back to the idea that numbers can be partitioned in multiple ways. This might become your learning intention, or essential learning goal for this time.
Relationships
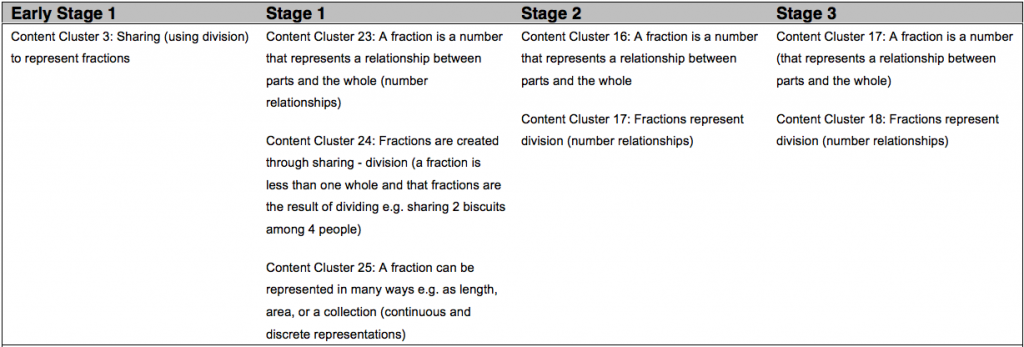
The second big idea is relationships. Students are exposed to many relationships between mathematical concepts for example, how two-dimensional shapes are the faces of three-dimensional objects, how possibilities in chance are related to fractions, how additive thinking and multiplicative thinking are related, how subtraction is the inverse operation to addition, the list goes on. Many of my clusters relate to relationships as a big idea, but one that students find particularly difficult is fractions as relational numbers. The first set of clusters above regarding partitioning pave the way for understanding fractions. One of the first models of fractions students are exposed to is part-whole. At a similar time, it is beneficial to introduce students to other models or concepts of fractions, particularly fractions as division (sharing) and fractions as a number. Students need to see multiple representations of fractions otherwise misconceptions develop early. If students are only exposed to the part-whole relationship they may rely on counting, this can lead to interpreting fractions as two whole numbers. The idea that 1/2 is one divided by 2 is important. As is the idea that 1/2 is a number, not two numbers, and that it has a place on a number line, just like whole numbers.
For the clusters I have developed in this group there are links to the following substrands:
- Early Stage 1 (Kindergarten): addition and subtraction, multiplication and division, fractions and decimals, two-dimensional space
- Stage 1 (Years 1 and 2): multiplication and division, fractions and decimals, length, time, two-dimensional space
- Stage 2 (Years 3 and 4): whole numbers, multiplication and division, fractions and decimals, angles
- Stage 3(Years 5 and 6): whole numbers, multiplication and division, fractions and decimals, patterns and algebra
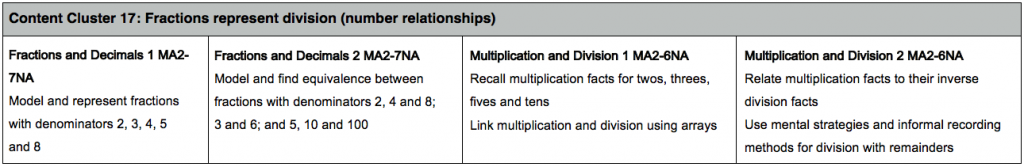
Again, in my planning I would select a cluster, if I was teaching a Year 3/4 class I might choose Cluster 17: Fractions represent division (number relationships). The cluster (image below) makes connections between fractions and division that can be visually represented in the array structure students may already be familiar with from learning about multiplication. Drawing on students’ prior knowledge about how to represent multiples using arrays, both with physical items and visually (say with dots), is a good launch into fractions as sharing or division. Students might be given opportunities to divide for example, a 4 x 5 array in half or quarters, labelling the parts using fractional terms (both in writing and numerically/symbolically). In these lessons, I would be addressing both the multiplication and division outcome as well as the fractions and decimals outcome. Tasks such as these would then be a great segue into creating equivalent fractions.
The examples above are just two big ideas and their related clusters. I would generally address approximately three clusters per term when business-is-usual! But in these times there is flexibility and more time to teach deeply. Although the pressure is off in ensuring every outcome is addressed this year, we still need to ensure our students are being expose to multiple concepts, and to concepts that matter most. I am in no way suggesting that this is all I would address this year, it is merely an example of how to ensure connections are made across aspects of mathematics while not addressing everything in its entirety. Clustering content under broader concepts not only allows you to draw on multiple substrands simultaneously, it allows you, and your students, the space to just sit within the mathematics. Keeping a learning intention or goal over a number of weeks means you can bring in other contexts in mathematics where the same concept is addressed. For example, when focusing on the concept of comparing and ordering, I can draw on content from length, area, volume, capacity and mass and teach them together, instead of writing five units individually and re-teaching comparing for each unit of measurement.
Are you interested in using the clusters at your school? I already know of several schools trying out my clusters in their school for planning and programming in mathematics. I’ve just set up a Facebook group for schools using them so we can chat about how they are going and for schools to share what they are doing. If you are already using them, or are interested in giving them a go, you can join the Facebook group here.
References
Mathematics K-10 Syllabus outcomes © NSW Education Standards Authority (NESA) for and on behalf of the Crown in right of the State of New South Wales, 2012.