I’ve been wondering recently about how to help students make connections between concepts and to their prior knowledge in the classroom. We know that mathematical ideas are connected and often build on each other, so how to ensure students ‘see’ these links? I think we have been moving in a good ‘direction of understanding’ in recent decades – talking in mathematics class is encouraged, and the talk has shifted from just the answer, to sharing the strategies students use. Kazemi and Hintz‘s book Intentional Talk has been instrumental in this change in my opinion, as has the work of both Pam Harris with #MathStratChat and Amanda Jansen with rough draft math.
Invite opportunities to revise in math class with #RoughDraftMath by @MandyMathEd. Explore how incorporating rough draft thinking into mathematics can make math more playful while helping students communicate, reflect & grow. #StenhousePub #StenhouseMath https://t.co/86MQTzpm0z pic.twitter.com/uBape3NxPg
— Stenhouse Publishers (@stenhousepub) November 2, 2022
After the Open Strategy Share, then what? In today’s #MathMonday blog, we highlight @ekazemi and @allisonhintz124’s Compare and Connect discussion structure from their book #IntentionalTalk. #StenhousePubshttps://t.co/Ga2iswI0Da
— Stenhouse Publishers (@stenhousepub) March 28, 2022
It's time for #MathStratChat! Rules: post your favorite or a clever solution! It's also fun to comment on other's strategies. Tell us about your reasoning. Like/Retweet so others can see! #MTBoS #ITeachMath #MathIsFigureOutAble #Elemmathchat #MSmathchat #HSmath pic.twitter.com/qUUF8gnDXv
— Pam Harris (@pwharris) October 27, 2022
I love the focus on students sharing their strategies, comparing their strategies with others, and justifying why their strategy works. But I’m wondering, how can we shift or extend the questions to focus on the mathematics itself, not the strategy or solution? Students need to be able to make statements about mathematics with a level or certainty or confidence, knowing something is true, and always true (or when it’s true), is the beginning of generalisation. I have been starting to use what did you find out? instead of what did you do? to have students focus on the mathematics itself after the lesson, but how can we start the lesson with this focus?
What do you know?
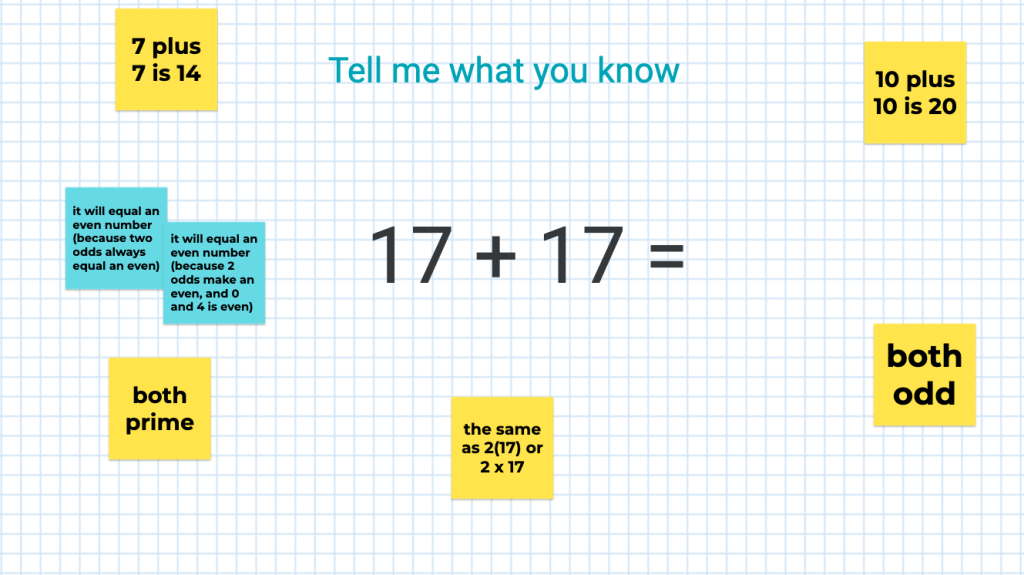
I’ve got an idea, which I’ve started experimenting with and I’d love other teachers to try it out and let me know how it goes. What if we focus on asking What do you know? If we want students to make connections to prior knowledge, we need to see if they can recognise known information in an unknown situation. Can they draw on what they already know that might help them solve the question? I want to get a list of ideas (or hints even) that might help us tackle the problem, without focusing on the procedural skills. Here are two examples I’ve tried. I put up the questions, stated I didn’t want the answer or how to solve the question, just what do you know [that might help us]?
I posed both of these ‘what do you know’ questions to a grade 6 and a grade 9 student. I had a list of ideas I was hoping to see (this builds on the anticipated strategies framework in Open strategy sharing from Kazemi and Hintz’s Intentional talk). I was pleasantly surprised with the ideas the students shared. They focused on the numbers themselves, the ‘oddness’ of 17 and what that might mean, they connected adding two identical numbers with multiplying by 2, they understood the place value of the digits, they identified decimals in the second question and produced a range which the answer might be within, and linked decimals to fractions.
The suggestions the students provided gave me evidence of what mathematical concepts they already knew, and which concepts they felt were important in answering these questions:
- number properties – odd and even
- doubles
- link between doubling and x 2
- place value – whole number and decimal
- relationship between fractions and decimals
- magnitude of numbers – how large the answer should be
Generalisations were already evident as well, with the statement:
‘it will equal an even number because two odds always equal an even”
It also showed clear links to prior knowledge of known facts such as 7 + 7 = 14, that will be useful in solving the first question. It also brought to light concepts that may need further discussion like the suggestion for the decimal question:
“it’s likely the answer is a decimal”
When probed further, the student stated that this ideas is “not always true”. This would allow me to probe in further lessons what possible ‘misgeneralisations’ students hold. I do not want to stop focusing on strategies, but for students to be able to choose the right strategy, and to be able to use knowledge in different situations or questions, then discussions focusing on what they [already] know are vital.
As part of mathematical proficiency, strategies must be intertwined with a range of other processes – importantly conceptual understanding. In NSW we talk about working mathematically as the thinking and doing of mathematics, I wonder if maybe it is also the knowing and doing?